The problem of the three cards

Description

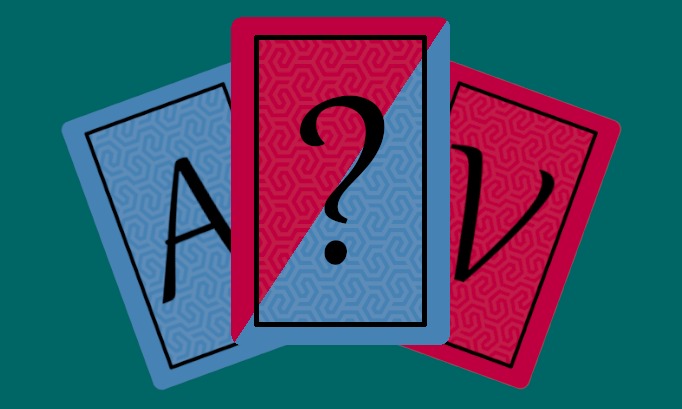
Consider that there are three cards, one with both red faces, another with both yellow faces, and a third with one red face and one yellow face. Knowing this information, a player draws one of the cards and sees only one side.

The question is:

What is the probability that the color of the hidden face is yellow/red?

The problem formulation often leads to the assumption that the probability would be ½ between the two possible cards. For example, if the yellow face is revealed, it may be assumed that the chance of it being the red-yellow card or the yellow-yellow card is equal. However, the misconception lies in the fact that the sample space was not considered correctly, in which each face of each card should be considered, rather than the cards in isolation.

How to make it at school/home

It is recommended to present the game of the three cards alongside the Monty Hall Problem given their similarity. The setup would be quite simple; dark-colored cards with color markings on each side can be used to configure the situation described above.

There is a variety of possibilities for applying this concept, meaning additional cards can be produced so that students can perceive the different probabilities according to the different combinations.

A proposed set of materials to be used:

• Coins printed with two identical sides (HH) and (TT), two distinct sides (HT). (H=heads, T=tails).
• Opaque cups where the coins would be deposited.

Learn more