Poincaré disk and Hyperbolic Geometry

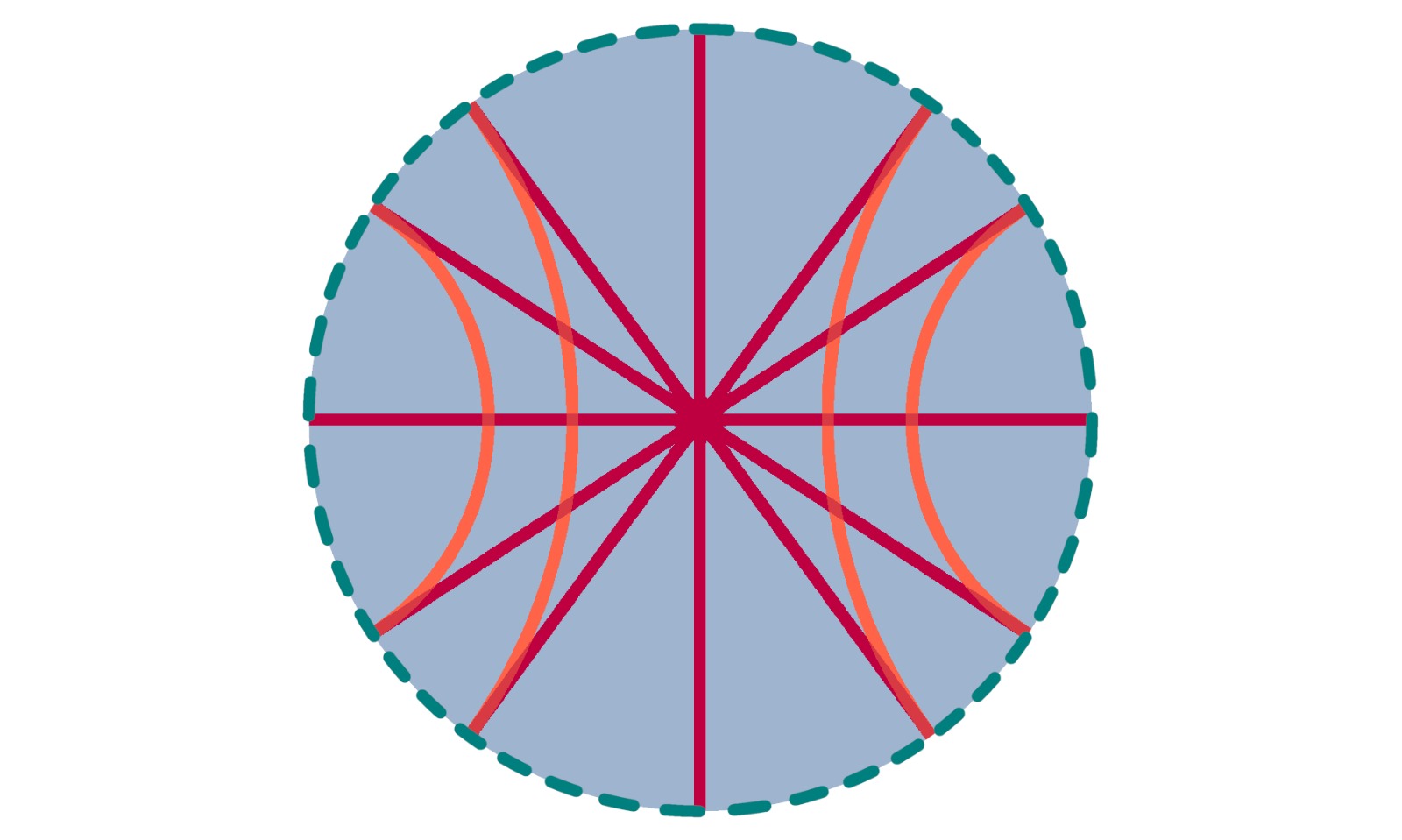
Historical Background

The Poincaré Disk is a visual representation of hyperbolic geometry developed by the French mathematician Henri Poincaré in the late 19th century. The motivation behind this representation arose from the quest to understand and explore non-Euclidean geometries, which were a paradigm shift from Euclidean classical geometry.

This activity has been designed with the purpose of introducing the  reader to non-Euclidean geometry through a visual and interactive  approach. Our goal is to assist the reader in comprehending the  unique properties of hyperbolic geometry, utilizing the Poincaré  Disk as a visualization tool. Hyperbolic geometry emerges from an  axiom where, given a point and a line in this space, there exist more  than one (infinite) lines passing through this point that do not  intersect with the provided line.

The Poincaré Disk played a fundamental role in understanding non Euclidean geometries by providing a visual representation for  hyperbolic geometry. The disk model, originally proposed by  Bernhard Riemann during a lecture in 1854 (which was later  published in 1868), gained more prominence through an article by  Eugenio Beltrami in the same year. However, it was Henri Poincaré  who incorporated the model into a comprehensive treatment of  hyperbolic, parabolic, and elliptic functions in his work of 1882.  Recognition of the model, however, came with Poincaré’s  philosophical work in 1905, “Science and Hypothesis,” in which  he introduced a conceptual universe now known as the Poincaré  disk. Within this region, space is described by Euclidean elements,  yet intriguingly, for inhabitants of this disk, the geometry is  hyperbolic, presenting a fascinating connection between the two  geometries.

Activity Description

Below, we outline the activities where we initially propose an  intuition about how distances behave distinctly for inhabitants of  the disk. Subsequently, we propose the construction of the Poincaré  disk.

Walkers in the Poincaré Disk

Consider inhabitants living within an area bounded by a  circumference. Something very peculiar happens to them: their legs  continuously shrink as they move away from the center of the  circumference. More specifically, consider that the circumference  has a radius R, and when the leg is at a distance r from the center  of the circumference, the length of the walker’s leg will be  proportional to R²-r² of its value at the center. For the walkers,  distances within this disk are measured by the number of steps they  need to move from one point to another.

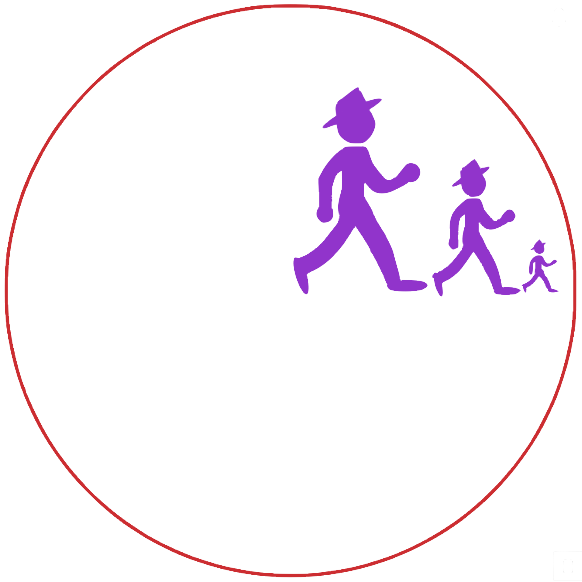
Challenges:

1. Given 2 points inside this disk, what are the paths on which  walkers take fewer steps between the points? In other words,  what are the paths of minimum length?

2. Consider a walker taking steps of size \frac{1}{2} when they start their  walk at the center of the disk with a radius R=1. How many  steps will it take for them to reach the boundary delimited by  the circumference? Would it be possible for a walker from  the disk to cross the boundary?

Hint: The Euclidean distance r_n from the center to the n -th step is given by r_n= \frac{3^n-1}{3^n+1}.

The above hint utilizes the fact that if r is the Euclidean distance  between the center and a point in the disk, then the distance for  inhabitants in the disk is given by ln(\frac{1+r}{1-r}), , which must be subsequently rescaled to the step size.

Construction of the Poincaré Disk (Do it at home/school)

We can construct the Poincaré disk by describing the behavior of  lines in space. As seen in the activity of walkers in the disk, the  distance from the center to the boundary will be infinite for the  inhabitants of this space. However, lines are defined by the shortest  paths in the disk, and, in a simplified manner, we will see how to  construct the shortest paths in this geometry.

In summary, the shortest paths will be lines that intersect the center  of the circumference and orthogonal circles, that is, arcs that belong  to circles whose tangents intersect perpendicularly to the boundary  (tangents) of the disk. The shortest paths are called geodesics.

Before we start the construction, let’s remember how we define the  inversion of points by a circumference.

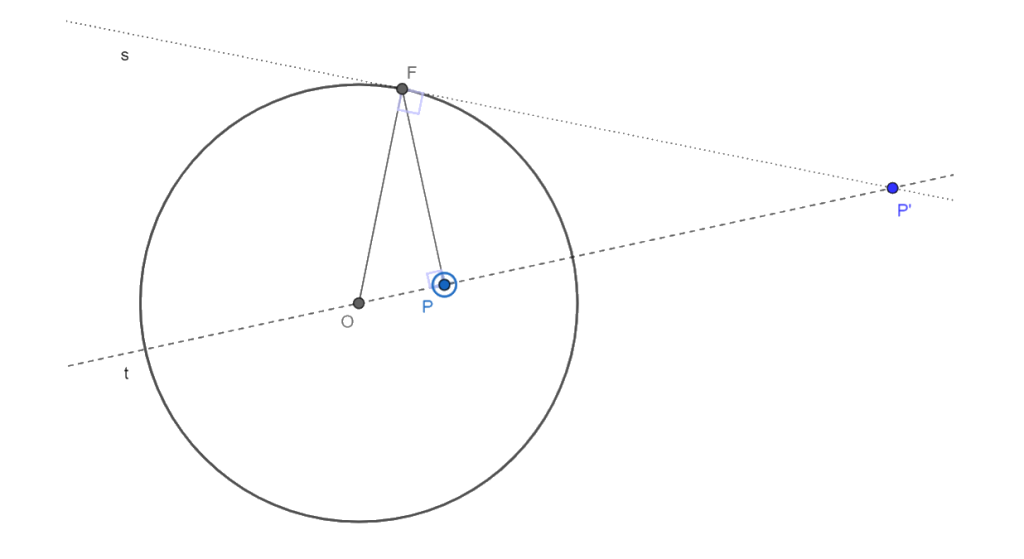
Point Inversion

• Let P be a point inside a circumference with center O, and let t be  the line containing O and P.
• Consider F to be a point on the circumference that belongs to the  line perpendicular to t intersecting point P.
• Let s be the line perpendicular to segment OF.
• The point P’ is the inversion of P by the circumference when P’ is  the point of intersection between the lines s and t.

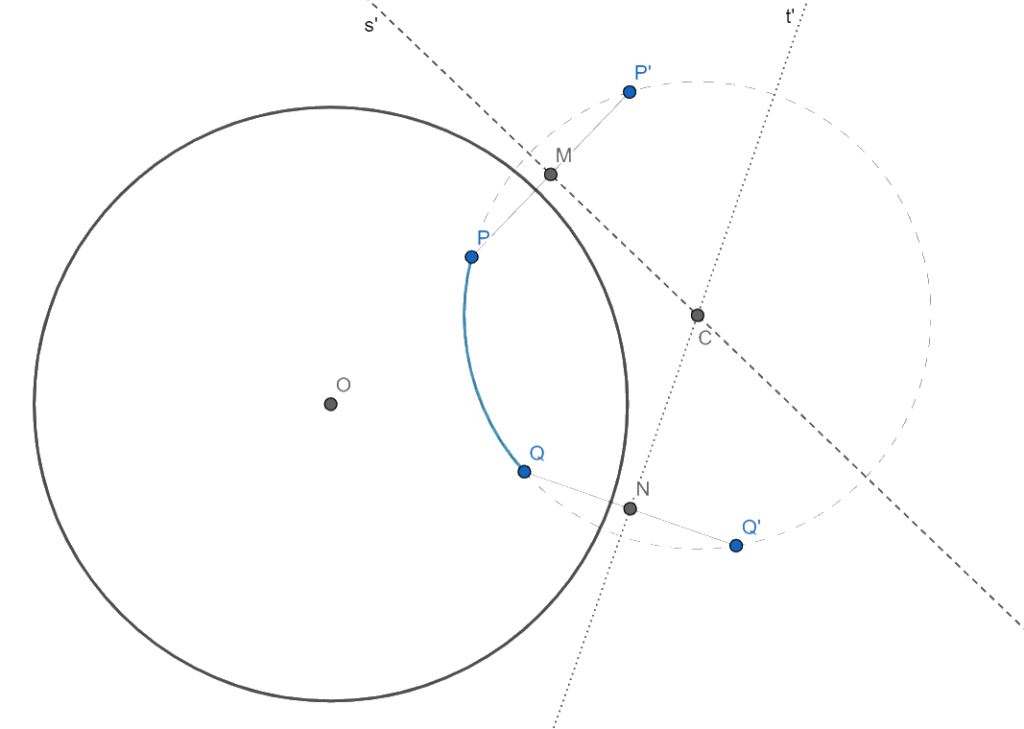
Paths in the Poincaré Disk

Consider P and Q as points inside the disk. Let l be the line  determined by P and Q. If O belongs to l, then the shortest path  between P and Q is the line segment PQ.

Otherwise, we perform the following construction using ruler and  compass:

• Let P’ and Q’ be the inversion of P and Q by the disk.
• Consider M and N as the midpoints of PP’ and QQ’, respectively.
• Let s’ and t’ be the lines perpendicular to PP’ and QQ’.
• Define C as the point of intersection between s’ and t’.
• The arc of the circumference between P and Q with center at C is  the geodesic between P and Q in the Poincaré disk.

Properties of the Disk

This disk allows us to verify several properties of hyperbolic  geometries. For example, we can construct a Khayyam-Saccheri  quadrilateral. Despite this quadrilateral having three right angles,  the fourth internal angle will be acute. Consequently, the sum of  the internal angles will be less than 2\pi.

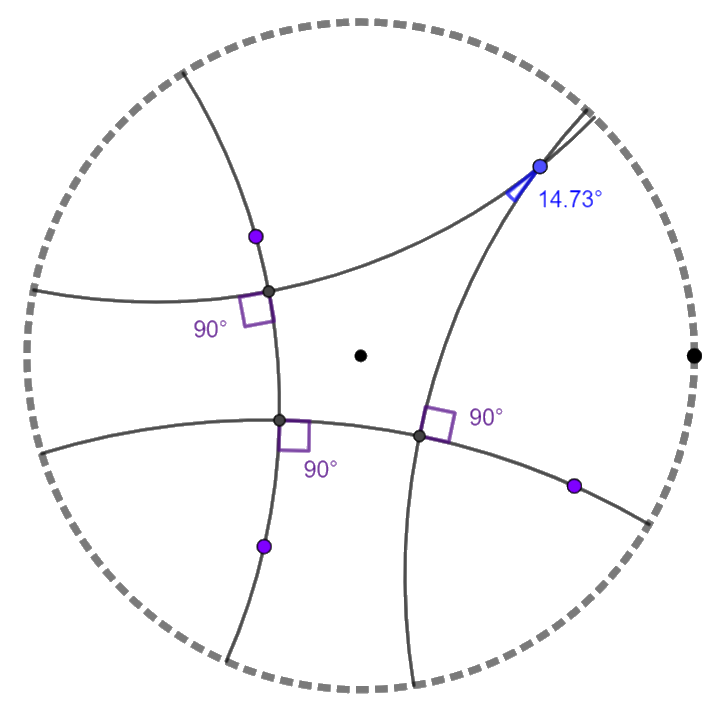
Since non-Euclidean geometry is based on disregarding the parallel  postulate, we have evidence of the existence of multiple parallel  lines between a line and a point.

Learn More

Explore more properties of hyperbolic geometry in the Poincaré  disk in the following application https://www.geogebra.org/m/mpzwcqka (provided by Guilherme A. Rink).

Another option would be to use a more constructive application  like the one available athttps://www.geogebra.org/m/R5e9AggU (in English)

In the following video, we can see a representation of the disk with  cars living in this space (instead of walkers), thus associating with  a projective property between a surface and the disk https://youtu.be/nu4mkwBEB0Y?feature=shared 

This model has also been explored in the arts, especially by Escher  in some works, see https://mathstat.slu.edu/escher/index.php/Circle_Limit_Exploration
If you are interested in learning more about the theory behind the  Poincaré disk with advanced mathematics (upper-level), we  recommend the text “Poincaré and his disk” by Étienne Ghys (in  English) https://perso.ens-lyon.fr/ghys/articles/Poincarediskenglish.pdf